The other irrational numbers we could celebrate instead of pi
Today is Mar. 14, or “Pi Day” (really, π Day) when mathletes and numberphiles celebrate the mathematical constant π, which is equal to 3.141592653…It’s a particularly special Pi Day because the year is 2015, bringing today’s date (3.14.15) two digits closer to π’s infinite decimal expansion.


Today is Mar. 14, or “Pi Day” (really, π Day) when mathletes and numberphiles celebrate the mathematical constant π, which is equal to 3.141592653…It’s a particularly special Pi Day because the year is 2015, bringing today’s date (3.14.15) two digits closer to π’s infinite decimal expansion.
But there are plenty of other infinite decimal (and infinitely useful) numbers to celebrate—Feb. 7 could have been e Day, for example, or Jan. 6, the Day of the Golden Ratio.
Part of π’s charm is its mix of measurable and undiscoverable: we know that π is exactly the ratio of a circle’s circumference to its diameter, and yet it is infinitely unknowable—the sequence of numbers following the decimal point in π has no pattern and goes on forever.
For all the digits of π that we can compute (the current record is about 12.1 trillion) and memorize (about 100,000) there will always be more beyond our grasp.
This is because π is an irrational number, which means it cannot be written as the ratio of two whole numbers.
Irrational numbers aren’t rare, though. In fact, there is what mathematicians call an uncountably infinite number of irrational numbers. Even between a single pair of rational numbers (between 1 and 2, for example) there exists an infinite number of irrational numbers.
So if we’re really going to honor mathematics on Pi Day, take a moment to mark a few more dates on the calendar, and discover the irrational numbers that equally deserve their own holidays:
e (pronounced “ee”)
Equal to about 2.71828182846…, e was discovered by Jacob Bernoulli, who first discovered it in a formula for calculating compound interest.
This mathematical constant also shows up in probability theory, number theory and calculus. Imagine playing a lottery with one-in-a-million odds of winning. The probability that you would never win—even if you played a million times—can be calculated with e: it’s about 1/e (less than 37%).
In the tech world, e already enjoys some celebrity—when Google filed for its first IPO in 2004, the amount they intended to raise was $2,718,281,828, or a billion times e.
φ (pronounced “phi”)
Equal to about 1.61803398875…, the irrational number φ is also known as the golden ratio or divine proportion. It is essential to geometry, and can be expressed as the ratio of a regular pentagon’s diagonal to the length of a side. Two numbers (say, a and b) are considered in the golden ratio if (a+b)/b = φ.
This ratio can also be derived from the Fibonacci sequence, in which each term is the sum of the previous two terms: 1,1,2,3,5,8,13,21,…The further along you go in the Fibonacci sequence, the closer the ratio of consecutive terms is to φ.
The irrational number φ has always fascinated mathematicians, astronomers, biologists and artists, since the ratio it represents, of course, is thought to have aesthetic appeal. Ratios close to φ can be found in Egyptian pyramids as well as in an abundance of architecture and artwork from the ancient Greeks and Mayans to the Gothic and Renaissance eras to modern pieces.
In the US, the German-American architect Mies van der Rohe’s Farnsworth House is said to be built according to φ.

√2 (pronounced “root two”)
Last and most venerable is the square root of 2 (written √2), which is equal to about 1.41421356237…Sometimes known as the Pythagorean constant, √2 is also the length of the hypotenuse of a right triangle, whose other sides both have length 1.
Likely the first number ever known to be irrational, legend holds that when the Pythagorean philosopher Hippasus showed his contemporaries a proof that the square root of 2 could not be expressed as a ratio of integers, around 500 BC, his peers were so shocked that they drowned him at sea.
Now of course, the discovery of irrational numbers is regarded as an important stepping stone towards modern mathematics.
There exist many different mathematical proofs of √2’s irrationality, including one so concise that it fits in a tweet:
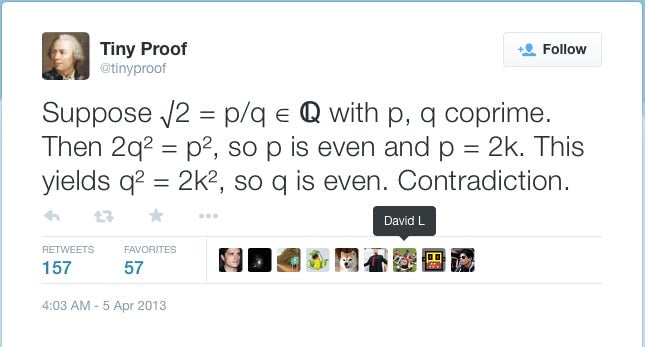
The elegance of brevity, for this first among irrational numbers, should be a healthy counterbalance to Pi Day’s lengthy recitations.